Tired of the Crookedness of the Financial System?
Q: What's the REAL underlying mechanism of Fractional Reserve Banking?
A: Preying on people's inability (or willful ignorance) to understand mathematics!

Once upon a time, Greek philosophers posed many problems which were of great interest in the day. Mathematicians now understand the literal interpretation of these questions as certain types of calculus problems. Modern-day philosophers, on the other hand, interpret each of these so-called paradoxes in metaphysical notions.
For our purposes, we will focus on the mathematical aspects of these philosophical questions, as they relate directly to the implementation of Fractional Reserve Banking.

Zeno's Paradox
A man is walking towards a destination and only moves halfway between his current location and where he's going. As such, the man must stop an infinite number of times before he proceeds to the next halfway point and, as such, will never attain his goal.
However, the distance between a man and his destination is finite. Surely, he will make it to his destination!
In fact, he does reach his goal in what is known as the limit or at infinity.
The Limit of Zeno's Paradox
Limits are notions that describe behavior in an aysmptotic sense, that is to say, in a general sense as some varying parameter approaches a particular value. The general rule is that one does not care what is happening precisely at the point that is being approached but rather is interested in the overall pattern in all possible paths that approach the destination.
I won't discuss the mathematics of limits (as one can abstract the notion further, especially when dealing with topological spaces), but the above description gives a decent picture. To reiterate: when limits are used, we do not care about the final limiting value, only in the qualitative behavior of all possible paths that approach the point of interest.
In the example where a man moves halfway from his current location to his destination, we can describe the infinite sum of all the finite distances that he moves in approaching his goal.
Infinite Sequences and Sums
(Since MathJax is not implemented, bear with me on not having decent mathematical notation.)
We describe this infinite sum by first considering the (finite) partial sum of all the first k steps.
First, we need to define the distance between the starting point and the end point to be some arbitrary value, a.
The distance that the man initially moves is a/2. The second distance is a/4, the third a/8, and so on. In fact, the distance of the k'th step of the man is a/(2^k).
Let us consider the sum of the first k distances. The first sum is a/2, the second sum 3a/4, the third sum 7a/8, and so on.
We write this as SUM(i=1..k, a/2^i) = S_k = (2{k}-1)a/(2k) .
If we take the limit of k as k approaches infinity, we see that the factor (2{k}-1)/2k approaches 1. In other words, if we say that M is a very large number, then M - 1 is also very large, and the ratio of (M - 1) / M becomes closer to 1 as M increases. Indeed, this is precisely the case when M = 2^k.
So you see, in the limit, the sum of the total distance traveled approaches a, which intuitively makes sense as that is the distance we defined between the start point and the end point.
A Picture is worth a 1000 words
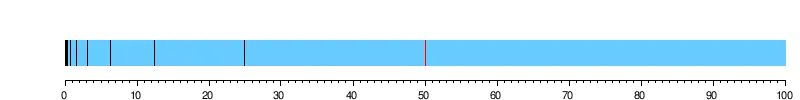
Here, 100 represents 100% of a and starting on the right, we move halfway towards 0. Near 0, we have an accumulation of points near the value we are approaching. In mathematics, we also say that a limit point is an accumulation point.
Infinite Geometric Series
The above example is nothing more than an application of an infinite geometric series.
An infinite geometric series is an infinite series of numbers where each value in the series is given by a (finite) partial sum. A partial sum is the sum of a finite set of numbers in a sequence. More generally, we write that the k'th finite value in an infinite sequence is given by
a_k = a r^{k},
where r is some geometric ratio that defines the change between one number in the sequence to the next.
The partial sum of the a_k's is given by s_k = SUM( i=0..k, a_k ) = a (1-r^k)/ (1-r).
The a_k's form a sequence and the s_k's form a series.
Without going into the details, s_k converges to a finite value as k approaches to infinity under the condition that |r| < 1.
This infinite sum converges to the value a / (1 - r).
Wutz Dis Gotta Due Wit' Moneez?
The mathematics of any monetary system that uses a fractional reserve policy is based entirely on infinite geometric series.
Ever heard of the money multiplier effect?
The lower the on-hand reserve requirement, the larger the money multiplier effect. Suppose a bank has a reserve requirement as low as 2.5%. Then, this equates to 39x increase in the monetary supply by doing nothing more than lending.

We first need to understand how a fractional reserve system operates. Then, we will see if we can spot the pattern of an infinite geometric series.
Ignoring all the intricacies of how the U.S. Treasury issues bonds in exchange for Federal Reserve Notes, suffice to say that someone (Congress) is doling out money to someone. So what's happening?
Say you are on the receiving end of Congress (although, normally, when I think of that phrase, I don't think of good things), and you are a contractor and manage to obtain a bid from the government to build a new bridge in the desert. Let's call your business, Business 0, and your bank, Bank 0.
You are appropriated x amount of Federal Reserve Notes. You take these notes and deposit them into Bank 0. Bank 0, being in the business of money lending, loans your money to a new business, Business 1, keeping only the minimum reserve requirement on hand. Business 1 receives the loan and places the money in Bank 1. Bank 1 creates a loan for Business 2 which in turn deposits the money in Bank 2. This process repeats ad infinitum.
What we are going to do is consider two different pairs of columns. One pair will be the two columns that contain the total sum that is loaned out by all the previous banks and the amount loaned out by the current bank. The other pair of columns will contain the sum of all the reserve money kept in all the previous banks and the reserve money kept in the current bank.
For simplicity, we will say that there is a reserve requirement of 10% and that the amount of money awarded in the contract bid was only $100,000,000. That is to say if we go back to our partial sums and infinite geometric series, we have that a = 10,000,000 and 90,000,000 for the Money Lent and Money in Reserve columns, respectively, and that and r = .9.
| Bank Number | Sum of Money Lent | Money Lent | Money in Reserve | Sum of Money in Reserve |
|---|---|---|---|---|
| 0 | 90,000,000 | 90,000,000 | 10,000,000 | 10,000,000 |
| 1 | 171,000,000 | 81,000,000 | 9,000,000 | 19,000,000 |
| 2 | 243,900,000 | 72,900,000 | 8,100,000 | 27,100,000 |
| 3 | 309,510,000 | 65,610,000 | 7,290,000 | 34,390,000 |
| 4 | 368,559,000 | 59,049,000 | 6,561,000 | 40,951,000 |
| ... | ... | ... | ... | ... |
| k | 90,000,000*(1-.9^k)/(.1) | 90,000,000*(.9)^{k} | 10,000,000*(.9)^{k} | 10,000,000*(1-.9^k)/(.1) |
You see that the columns correspond to a two different pairs of geometric sequences and series.
Observe that the total amount of money held in reserve by all the banks as we approach infinity (and note that only after 4 iterations, we are almost halfway there) is the initial amount for the original contract, i.e. $100,000,000. But, wait, there's all that money that is loaned out to people now. There is $900,000,000 of that funny money floating around for only $100,000,000 kept in the banks.
The money multiplier effect can be calculated by taking (1 / reserve requirement %) - 1. For banks, who have a reserve requirement of 10%, we see that the money multiplier effect is 9 times.
Fun fact: Canada, the UK, New Zealand, Australia, and Sweden have no reserve requirement!
This is how a fractional reserve banking system creates money from nothing. Notice that we didn't even have to use usury (charging interest on loans!) to create new currency.
