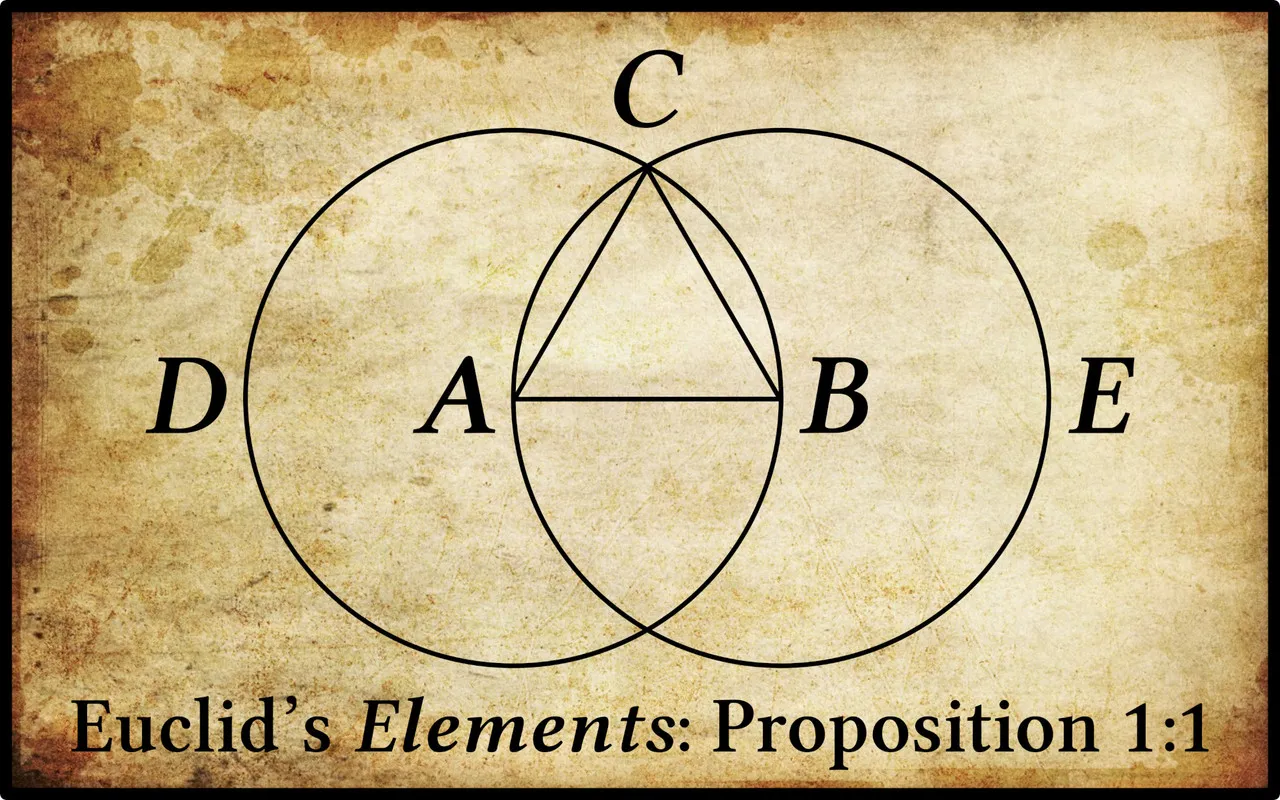
Book 1 of Euclid’s Elements opens with three constructions. The first of these, Proposition 1:1, demonstrates how to construct an equilateral triangle:
| Greek | English |
|---|---|
| αʹ. Ἐπὶ τῆς δοθείσης εὐθείας πεπερασμένης τρίγωνον ἰσόπλευρον συστήσασθαι. | 1. To construct an equilateral triangle on a given finite straight-line. |
Euclid―or one of his folowers (Joyce)―defined triangles in Definitions 19 and 20:
19. Rectilinear figures are those (figures) contained by straight-lines: trilateral figures being those contained by three straight-lines, quadrilateral by four, and multilateral by more than four.
20. And of the trilateral figures: an equilateral triangle is that having three equal sides, an isosceles (triangle) that having only two equal sides, and a scalene (triangle) that having three unequal sides. ―Fitzpatrick 6
But do equilateral triangles exist? By demonstrating how to construct an equilateral triangle, Euclid proves that they exist. Expressed in modern language, this proposition and its demonstration run as follows:

The Formal Divisions of a Proposition
In manuscripts of Euclid’s Elements each proposition is generally written as a continuous block of text, without any headings for the different parts of the theorem. But it was customary to divide a proposition into six formal parts, as Proclus describes in his Commentary:
Every problem and every theorem that is furnished with all its parts should contain the following elements: an enunciation, an exposition, a specification, a construction, a proof, and a conclusion. Of these the enunciation states what is given and what is being sought from it, for a perfect enunciation consists of both these parts. The exposition takes separately what is given and prepares it in advance for use in the investigation. The specification takes separately the thing that is sought and makes clear precisely what it is. The construction adds what is lacking in the given for finding what is sought. The proof draws the proposed inference by reasoning scientifically from the propositions that have been admitted. The conclusion reverts to the enunciation, confirming what has been proved. ―Morrow 158
Proclus’s Greek text provides the technical terms by which these six parts are known, though the English translations of these vary from source to source:
| Greek | English |
|---|---|
| πρότασις | Proposition, Enunciation |
| ἔκθεσις | Exposition |
| διορισμός | Definition, Specification, Determination |
| κατασκευή | Construction, Machinery |
| ἀπόδειξις | Proof, Demonstration |
| συμπέρασμα | Conclusion |
Proposition What is given and what is to be proved.
Exposition What is given.
Definition What is to be proved.
Construction Completion of the geometric diagram.
Proof Logical proof of the proposition.
Conclusion A restatement of the proposition, which has now been proved.
Not all parts are required, as Henry Billingsley explains in his 1570 edition of the Elements:
Moreover every Probleme & Theoreme, beyng perfect and absolute, ought to have all these partes, namely: First the Proposition, to be proved. Then the exposition which is the explication of the thing geven. After that followeth the determination, which is the declaration of the thing required. Then is set the construction of suche things which are necessary ether for the doing of the proposition, or for the demonstration. Afterward followeth the demonstration, which is the reason and proofe of the proposition. And last of all is put the conclusion, which is inferred & proved by the demonstration, and is ever the proposition. But all those partes are not of necessitie required in every Probleme and Theoreme. But the Proposition, demonstration, and conclusion, are necessary partes, & can never be absent: the other partes may sometymes be away. ―Billingsley Folio 8
In his 1826 edition of the Elements Ernst Ferdinand August includes Proclus’s technical terms―or equivalent terms―before the relevant sections of Proposition 1:1, but for the remaining propositions he only includes the initial heading Πρότασις. François Peyrard also follows this practice in his edition of 1814–18. Apparently this practice was also followed by three of the manuscripts Peyrard consulted: Codex Vaticanus Graecus 190 (9th–10th century), Codex Parisinus Graecus 2466 (12th century) and Codex Parisinus Graecus 2344 (12th century). In the following image from Vat Gr 190 each of the six technical terms has been placed in a black cartouche:

When Johan Ludvig Heiberg & Heinrich Menge brought out their definitive edition of the Elements in 1883, they chose to omit all such headings, considering them to be non-authorial. Even the word πρότασις is omitted, each proposition being preceded only by the appropriate Greek numeral.
Commentary
With the exception of the Pythagorean Theorem (Proposition 1:47), more ink has probably been spent on this proposition than any other in the Elements. Its place of honour at the head of Book 1 has lent it a reputation it hardly deserves. The most frequent comment concerns Euclid’s assumption that the two circles must intersect:
The assumption that the circles do indeed cut one another should be counted as an additional postulate. ―Fitzpatrick 8
It is a commonplace that Euclid has no right to assume, without premising some postulate, that the two circles will meet in a point C. To supply what is wanted we must invoke the Principle of Continuity ... ―Heath 242
It might be argued that the intersection of the circles is obvious from the diagram. Perhaps this is why Euclid omits to include this assumption among his postulates. But we must recall that these drawings are not circles: they are marks on a page that represent circles. Real circles are abstract ideas. They cannot be seen with the eyes. They can only be apprehended by the mind. Imagine that you have been charged with teaching Euclidean geometry to someone who was born blind. You are forbidden to use diagrams or Braille. You must teach the subject from an entirely abstract perspective. Under these constraints, it is no longer so obvious that the circles must intersect.
In Proposition 3:10 Euclid proves that a circle does not cut another circle at more than two points, so he is not entitled to assume this here.
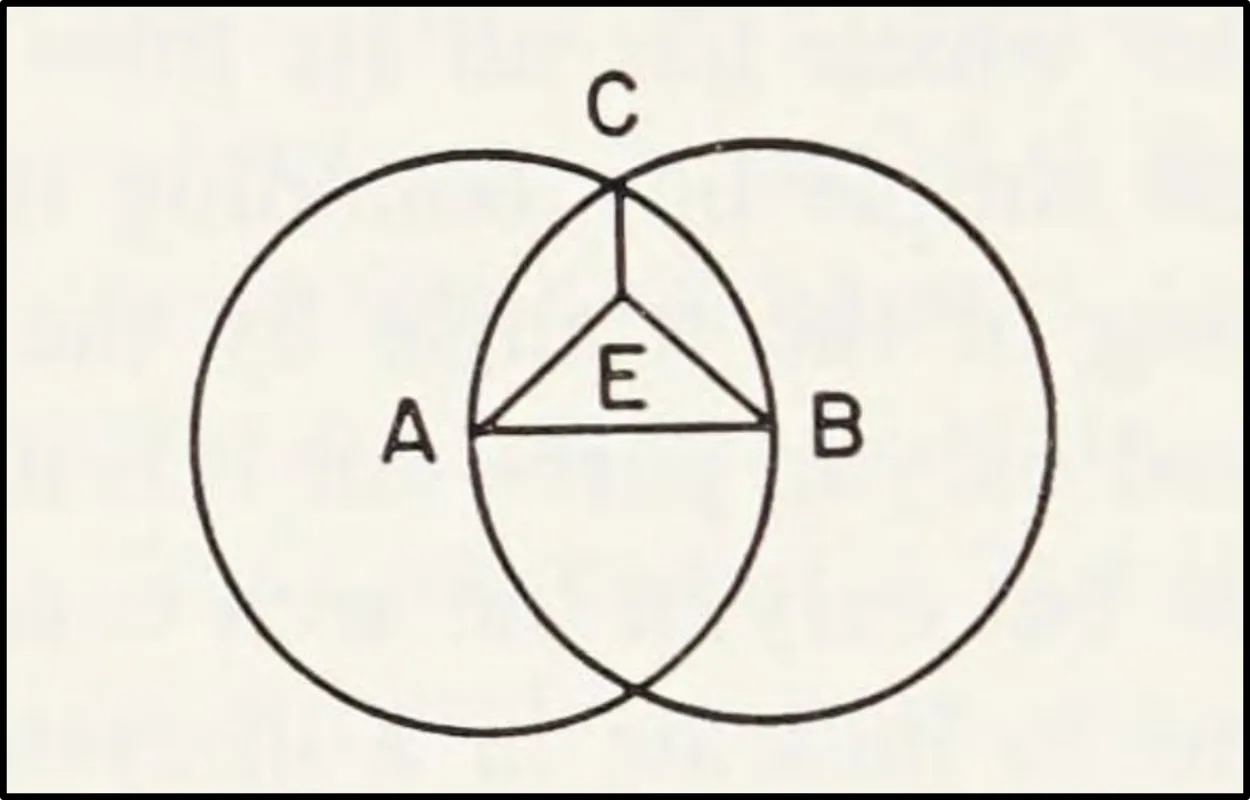
Fitzpatrick also points out another omission in Euclid’s demonstration:
There is also an implicit assumption that two straight-lines cannot share a common segment. ―Fitzpatrick 8
The Epicurean philosopher Zeno of Sidon was the first to point this out. Zeno was a contemporary of Cicero’s, who attended some of his lectures in Athens in 79 BCE. Only a few fragments of his copious writings are extant. It is thanks to Proclus that his criticism of Euclid’s first proposition has survived:
Since some persons have raised objections to the construction of the equilateral triangle with the thought that they were refuting the whole of geometry, we shall also briefly answer them. The Zeno whom we mentioned above asserts that, even if we accept the principles of the geometers, the later consequences do not stand unless we allow that two straight lines cannot have a common segment. For if this is not granted, the construction of the equilateral triangle is not demonstrated. Let AB be the straight line, he says, on which we are to construct the equilateral triangle. Let the circles be drawn, and from their point of intersection draw the lines CEA and CEB having CE as a common segment. It then follows that, although the lines from the point of intersection are equal to the given line AB, the sides of the triangle are not equal, two of them being shorter than AB. But if their equality is not established, neither are its consequences. Therefore, says Zeno, even if the principles be granted, the consequences do not follow unless we also presuppose that neither circumferences nor straight lines can have a common segment. ―Morrow 168
In Proposition 11:1 Euclid invokes this very axiom, calling it impossible for two different straight lines to share a common line segment (Fitzpatrick 425). But this was not Zeno’s only criticism of this proposition:
Proclus devotes considerable space to this part of Zeno’s criticism, but satisfies himself with the bare mention of the other part, to the effect that it is also necessary to assume that two circumferences (with different centres) cannot have a common part That is, for anything we know, there may be any number of points C common to the two circumferences ACE, BCD. ―Heath 242

Vincenzo De Risi
Recently the Italian historian of philosophy and science Vincenzo De Risi has explored the theory of geometric intersections and its significance to the foundations of mathematics:
As is well known, at the end of the nineteenth century the mathematician Moritz Pasch criticized Euclid for having assumed without justification the existence of the points of intersections between the lines and circles that he traced in his constructions. Pasch pointed his finger at the very first proposition of the Elements, the one showing how to construct an equilateral triangle on a given segment ... Euclid had been careful in spelling out the assumptions needed to perform such a construction ... Yet, he overlooked (or so claimed Pasch) a most important assumption, viz., that the two crossing circles do indeed intersect one another, so that their point of intersection exists. Pasch accused Euclid of relying on his eyes rather than upon his mind, pretending that he was to see in the diagram the existence of such a point. In Pasch’s mind, however, the foundation of mathematics demanded stricter, propositional standards of rigor: the task of modern axiomatics, Pasch said, consisted in filling this and like gaps in Euclid’s proofs, by introducing to this effect all the necessary axioms. ―De Risi 12–13
De Risi’s paper on this chapter of mathematical history is available online.
Finnegans Wake
As a Dubliner who keeps a blog on James Joyce’s final novel Finnegans Wake, I could hardly discuss Euclid’s Proposition 1:1 without at least mentioning its appearance in Finnegans Wake. Joyce drew upon this theorem for the school lessons of brothers Dolph and Kev (Shem and Shaun) in Chapter II:2:
Problem ye ferst, construct ann aquilittoral dryankle. Probe loom! With his primal handstoe in his sole salivarium. Concoct an equoangular trilitter. On the name of the tizzer and off the tongs and off the mythametical tripods. Beatsoon. ―Joyce 221 (The Restored Finnegans Wake 221.17–20)
What is a very dry mathematical problem in Euclid is charged with sexual overtones in Finnegans Wake. Euclid’s triangle becomes a river delta, Δ, the symbol for their mother ALP (Anna Livia Plurabelle). The overlapping of the two circles creates a vesica piscis, which also carries much symbolical meaning. But this is hardly the place to pursue such scandalous matters. The curious reader can read Sangam Macduff’s chapter on the subject in Volume 29 of the European Joyce Studies series.
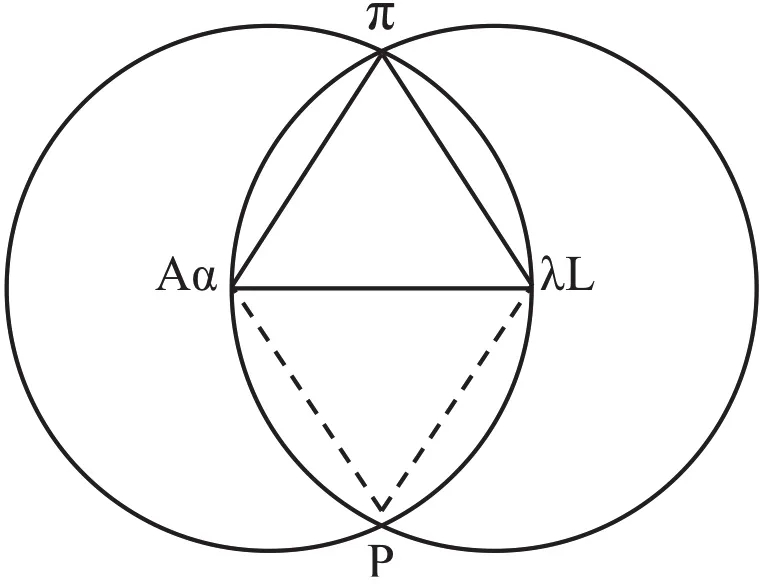
Euclid’s famous diagram―slightly altered―appears on page 226 of The Restored Finnegans Wake (page 293 of the original edition of 1939), approximately halfway through the book. Symbolically, it represents the centre of the book, which is circular. The opening sentence of Finnegans Wake is really only the second half of the closing sentence, turning the work into a literary ouroboros.
And that’s a good place to stop.
References
- Ernst Ferdinand August, Euclidis Elementa, Part 1, Theodor Trautwein, Berlin (1826)
- Henry Billingsley (translator), The Elements of Geometrie of the Most Auncient Philosopher Evclide of Megara, John Day, London (1582)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1–5, B G Teubner Verlag, Leipzig (1883–1888)
- James Joyce, Finnegans Wake, The Viking Press, Twelfth Printing, New York (1966)
- James Joyce, The Restored Finnegans Wake, Edited by Danis Rose & John O’Hanlon, Houyhnhnm Press, Dublin (2010)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Sangam Macduff, The Logic of the Doodles in Finnegans Wake, Emily Louise Silva et al (editors), James Joyce and the Arts, European Joyce studies, Volume 29, Pages 166–180, Brill-Rodopi, Leiden (2020)
- Glenn Raymond Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, New Jersey (1970)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1–3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
- Vincenzo De Risi, Did Euclid Prove Elements I, 1? The Early Modern Debate on Intersections and Continuity, P Beeley, Y Nasifoglu, B Wardhaugh (editors), Reading Mathematics in the Early Modern Europe: Studies in the Production, Collection, and Use of Mathematical Books, Pages 12–32, Routledge, London (2020)
- Thomas Taylor, The Philosophical and Mathematical Commentaries of Proclus on the First Book of Euclid’s Elements, Volume 1, Volume 2, London (1792)
Image Credits
- Vaticanus Graecus 190 (Folio 15r): Vatican Apostolic Library, Vatican City, Public Domain
- Vincenzo De Risi: © Université Paris-Cité, Fair Use
Online Resources
